Based on empirical research work from his PhD thesis on electoral system in plural societies, Rama Sithanen emphasizes the need to thoroughly understand the mathematics of voting formulae before making recommendations on the White Paper...

The aim of this paper is to explain, in simple terms, the mathematics of electoral reforms. A moderate knowledge of mathematics is required to evaluate the consequences of reform proposals as the mathematical formula used has significant impact on stability, party fairness, rainbow and gender representation and the eligibility thresholds. It may even determine the balancing act between being fair to small parties and the necessity to preserve national unity and social cohesion in a multi-faith and multi-ethnic society. It is a fact that some well-intentioned stakeholders have difficulties to grasp the significance of some formulae, as applied to electoral reforms. This invariably leads to misgivings, confusion, ill–informed judgement and wrong choice. The country must make sure that it does not ignore mathematics at its own risks. I shall attempt to throw some light on three issues that are of key importance for electoral reform. The analysis is based on empirical research work that I carried out for my PhD thesis on electoral system in plural societies. It was important to thoroughly understand the mathematics of voting formulae and their implications before making recommendations on the choice of electoral system.

Of the eleven proposals contained in Chapter 10 of the White Paper on electoral reform, there are few being debated robustly, albeit one or two more than others in terms of passion and intensity. These include how many PR seats (from at least 16 to 20 ) is necessary to complement the existing FPTP MPs, the number of PR doses (from one of, at least, 16 seats to two doses of 28 seats), the method of returning these Party List MPs (from a pre-polling, closed and rank-based Party List to being chosen after the elections by Party leaders or a combination of the two) and the eligibility threshold for such PR seats (5%, 7.5% or 10% of national votes). There is also a commendable disposition between the two main parties to discuss and negotiate so as to whittle down their differences and to reach consensus on the way forward. It is indeed true that we have never been so close to an agreement on electoral reform and we must seize this unique opportunity with both hands. However it is imperative that we understand the consequences of each of the options on the table. As there is no perfect electoral system, one would expect people to differ widely on what criteria matter most when choosing a voting formula. Their analyses are based, rightly or wrongly, on what they believe is good for the country. That is why there are so many electoral systems around the world.

There is however one area where there is a hell of unnecessary confusion as the issues are fact-determined and evidence-based and absolutely not value-driven or idea-related. This concerns the mathematical formulae that should be used to ascertain the number of PR seats that will go to each eligible party, the confusion between a mixed member proportional system and a full PR formula and the implications of legal and natural eligibility thresholds to obtain PR seats.

In the current debate, there are at least three huge sources of pandemonium on PR and the mathematics of its applications.

i) When people believe that two altogether different concepts are similar. From a standard political and electoral science standpoint, there are two ways of alloting PR seats, which are the parallel mode and the compensatory mode. Both face challenges in their implementation. Many do not make the very simple difference between a parallel formula (Sachs A) and a compensatory method (Sachs C) of apportioning PR seats. As a result, much time is wasted as people honestly believe they are speaking about the same thing when in fact they are talking at cross purposes because of a lack of understanding. And it poisons the debate irrationally and stupidly as it is clearly at odds with hard facts and empirical figures. Simple arithmetic should enlighten the debate ;

ii) When people think that two similar concepts are in fact different. Almost everybody seems not to recognise that there is no difference between a mixed electoral system (FPTP and PR) as recommended by Sachs C and a full proportional system of only PR seats as contained in Sachs D and in Carcasonne. I must acknowledge that this is not obvious to the naked eyes and those not very familiar with the theory and practice of electoral systems. As a consequence, there are many well intentioned and arguably very intelligent people who are opposed to a full PR voting formula and yet want to opt for the compensatory method of Sachs C to allocate PR seats in a mixed electoral system. And they fail to realise that the outcome is indeed a full PR system with the share of votes matching accurately the share of seats. Exactly what they do not want in the first instance. Obviously this would be at the expense of stability, governability and decisiveness. This confusion too is clouding the debate unnecessarily and unwisely. Plain mathematics should help to fill this knowledge gap ;

iii) Some actors, acting in good faith, have suggested that there is no need for a legal threshold for parties to be eligible for PR seats. According to them, with 20 PR seats, the system will simply ensure that parties are allocated one seat per each 5 % of national votes. This is what is called a ‘natural threshold’. Thus a party that polls 5% of votes will be entitled to one PR seat. This magic figure is obtained by dividing 100 into 20 seats. It looks so easy and so beautiful. Unfortunately it is not correct. This innocent proposal hides a monumental blunder that could cause untold damage to the reform process. I shall also, in the process, consider the consequences of a legal threshold of 5% as argued by some stakeholders, in all good faith, so as to give voice and representation in Parliament to small parties. Again the mathematics of electoral reform can help break this logjam. Decisions should be made in full cognisance of their known consequences.

The members of Parliament have never been so close to an agreement on electoral reform. © DEV RAMKHELAWON

Right from the outset, it should be very clear that there are two main reasons underpinning reform of the voting formula. First is the necessity to cure the very high disproportionality between seats won and votes polled which leads to the significant underrepresentation of some parties. And second is the need to remedy the amplification of ‘winner takes all’, where one alliance could obtain 100% of seats with around 51% of votes, thus leaving no elected representation at all to other parties with a relatively high share of votes. This can be done by introducing a greater degree of fairness, measured by a better distribution between seats and votes, in our electoral system. However in so doing, we must make sure that the stability, governability and decisiveness of the system is not undermined. The challenge is whether we can devise an alternative voting system, which while alleviating the existing problems, will not create or worsen others. Of course, in the ensuing discussion, it was important to take on board the issue of gender fairness and the obligation to find an acceptable solution to the legal challenges posed by the Best Loser System.

Confusion One : Many think that Sachs A and Sachs C are the same. They are very different.

While many grasp the difference between FPTP and PR systems, few make the fundamental distinction between a parallel PR (Model A of Sachs) and a compensatory PR (Model C of Sachs) of allocating PR seats, when used in tandem with FPTP. A parallel PR mode returns candidates in complete disregard of constituency elections while the compensatory PR determines the apportionment of PR seats while taking into account the constituency outcomes. A parallel PR does not recognise ALL the votes cast in BOTH modes in alloting the PR seats. It could thus lead to huge disparities between overall votes and total seats, thus defeating the main purpose of the reform which is to narrow the disproportionality between share of votes and share of seats. I meet many people who would like to have a fair system but still choose a parallel formula. This is a mathematical contradiction.

By contrast, constituency seats won are taken into account in the compensatory PR. The method aims to align the total seats (constituency plus PR) of each party to its votes. A party which has obtained more constituency seats than warranted by its share of votes will receive fewer or no PR seats. It is in fact a highly proportional system. The issue of fairness of representation has been the centrepiece of all reports on electoral reform in Mauritius. The parallel formula was found to be very insignificant in correcting the unfairness emanating from the FPTP system as it leaves huge distortion between seats and votes. The presence of the Opposition would be symbolical only and it would not be able to play a meaningful role in Parliament. It should be noted that Sachs and other experts had no hesitation whatsoever to reject the parallel formula. It was not even ranked second after the preferred choice of the experts for the compensatory mode (Model C). As a matter of fact Model B of Sachs was considered much superior to Model A. All electoral specialists are categorical against the parallel formula because of its very limited impact on fairness.

However the compensatory mode of allocating PR seats could convert the electoral system into a full proportional one in many cases and thus make it extremely difficult, if not impossible, to have effective government. This will happen at the expense of stability, governability and decisiveness.

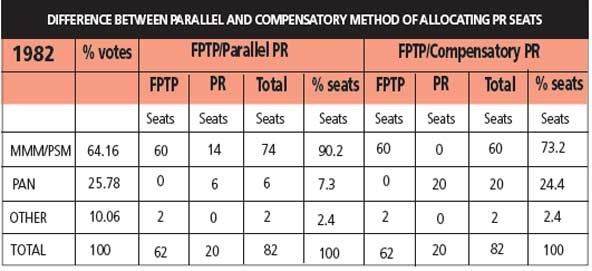
The table below gives the significant difference between the parallel and the compensatory modes for the elections of 1982 in Mauritius. Even if some genuinely think they are similar, the two formulae are absolutely different .

In 1982, the parallel mode, which disregards the fact that PAN did not receive a single seat for 25.78% of the votes in FPTP elections, would have allocated only 6 seats to the second party and 14 to the winning alliance, based simply on their respective share of votes, after eliminating parties that do not meet the threshold to be eligible for these PR seats. The final outcome would have been 74 seats for the MMM/PSM and 6 seats for PAN. It is highly disproportional with PAN attracting only 7% of seats for 25.78% of votes and the MMM/PSM capturing 90% of the seats on 64% of votes.

The compensatory method recognises the unfairness of the FPTP elections and ‘compensates’ PAN for its share of votes using the D’Hondt formula. Instead of receiving a share of the 20 PR seats only, PAN is entitled to a percentage of the total number of seats (FPTP plus PR). This makes a major difference in seat allocation. PAN would have received all 20 PR seats as it was severely penalised in the constituencies. The final result would be 60 seats for MMM/PSM (no PR seat at all) and 20 seats for PAN. The second party thus obtains 24.4% of seats for 25.78% of votes. It is almost a proportional outcome. The small difference between the two figures is the impact of thresholds. The parallel formula is very insignificant in correcting the unfairness of the system. Worse it widens the difference in the number of seats between the two parties from 60 to 68!

It is plain that if the main objective of reform is to correct the gross under-representation of opposition parties produced by FPTP, then the parallel mode has a very marginal impact and does not provide for a reasonable measure of proportionality. And it should rightly be rejected as a formula to allot PR seats. But then should we go to the other extreme of ensuring perfect proportionality in the electoral system by using Model Sachs C? And throw away the baby of stability and effectiveness with the bathwater. Extremes could be very bad! Too much proportionality also is not a desirable feature.

Dr Sithanen continues today with the mathematics of the electoral reform following publication of Part I yesterday. He proposes the use of an alternative formula to allocate PR seats among eligible parties, based on ‘Wasted Votes’. Which is not an ideal formula but is much better in terms of fairness and stability he says.

Confusion Two: Many believe that Sachs C and Sachs D are different. They are the same.

Many well intentioned stakeholders do not always differentiate between a mixed system as recommended by Sachs C and a full, stand alone, PR as contained in Sachs D. The compensatory formula of the mixed system advocated by Sachs C is similar to what exists in Germany, New Zealand and Lesotho. It is in fact a proportional system even if it looks like a mixed model. Therein lies the confusion. As a matter of fact, its correct terminology in the political literature is a Mixed Member Proportional system. If the number of seats in the PR component is relatively high and the threshold of eligibility low, then the compensatory mode of Sachs C becomes a pure PR model. And stability, governability and decisiveness will be threatened.

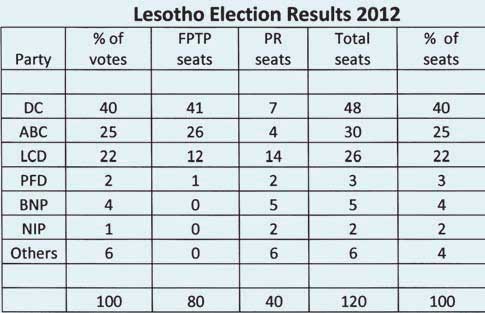
I shall use the example of Lesotho to illustrate the point. Lesotho is a SADC country with a small population base, not dissimilar from us. It inherited, just like Mauritius, the British system of FPTP until it changed to a mixed FPTP/ PR formula using the compensatory mode of Sachs C to allocate seats. Since 2002, Lesotho has a Mixed Member Proportional system, similar to Germany and New Zealand. Each voter has two votes and there is no legal threshold. The first vote is a personal one in 80 FPTP constituencies. The second vote is for the party with 40 PR seats. Thus, Lesotho, on paper, operates a mixed system with 80 FPTP seats (two third of the total of 120 seats) and 40 PR seats (one third of the 120 seats). Candidates are allowed to compete in FPTP districts as well as simultaneously for the party list. The candidates who achieve a plurality of votes in the single-member districts are elected. However, it is the National Party vote that determines how many representatives will be sent from each party to Parliament. As a result, all the party votes are totalled and their share of seats ascertained. Then the number of seats won directly by a party in the FPTP constituences are substracted from the total number of seats allocated to that party. The remaining seats, if any, are then assigned to the closed, rank-based party list, published ahead of elections.

Because of the compensatory formula used to allot seats, the system is not, as often believed, a mixed one, but a pure PR system. Especially if the number of PR seats is relatively high and the threshold of eligibility low. There are, however, two differences between the two systems. First the personal vote for a candidate in FPTP constituencies ensures a close relationship between voters and their representatives. Second, if there are not enough PR seats to compensate the second, third and other parties, there may still be some distortion between votes polled and seats won. However, in the majority of cases, the final outcome would be a highly proportional one that is identical to what would happen under Sachs D with a full PR system. This is shown in the table below.

There are two simultaneous elections (one FPTP and one PR) but they are inextricably linked as what happens in one mode affects the election in the other one. The main observations from the 2012 election results of Lesotho are extremely instructive for Mauritius as it embraces reform.

i) The DC party took 41 of the 80 FPTP seats while ABC captured 26 and LCD obtained 12 of those seats. One seat went to the PFD party; 

ii) It should be noted that if Lesotho had kept its FPTP formula, DC party would have won an absolute majority with 41 of the 80 seats and formed the government ; 

iii) The DC party polled 40% of the National votes while ABC and LCD garnered 25% and 22% respectively. Around 10 other small parties shared the remaining 13% of the votes; 

(iv) The compensatory mode of allocating the PR seats means that those parties disadvantaged by the FPTP contests are ‘rewarded’ in the apportionment of the 40 PR seats. This is reflected by the fact that LCD party and the other small ones took the lion share of these PR seats; 

(v) As the winner of the FPTP contest took more than 50% of these seats on only 40% of the votes, it received very few PR seats. Equally for Party ABC which captured more than its share of constituency seats; 

(vi) Since it is a highly proportional system, the share of seats is identical to the share of votes. Overall, the DC party gained 40% of the seats on 40% of the votes; similarly ABC party won 25% of the seats with 25% of the votes. And similarly for the other parties; 

(vii) Had Lesotho opted for a full proportional voting system (Sachs D) instead of a mixed member proportional (Sachs C) , the outcome would have been identical. If there were 120 list PR seats to be allocated, a party such as DC with 40% of the votes would have taken 40% of the 120 seats. This is equal to 48 seats, exactly the same number of seats as under the FPTP/PR formula. Absolutely no difference. This is not even tantamount to introducing a full PR through the backdoor. It is entering the main gate and taking the most important seat at the table; 

(viii) By producing highly proportional outcomes, the mixed FPTP/PR electoral system can produce unstable governments as was the case in Lesotho in 2012. Hung Parliaments mean that coalition governments are required, arduous and protracted negotiations necessary and alliances can change quickly. As expected in such a system, there is no overall winner. Stability pays an extremely heavy price for proportionality. The largest party, DC, has only 48 seats out of a total of 120. From DC party being a clear winner after the FPTP contest, the compensatory formula delivers a hung Parliament overall; 

(ix) The political and party system is hugely dispersed. From four parties that won FPTP seats, Lesotho ended up with 12 parties having seats in Parliament. This is proportionality at the expense of political fragmentation ; 

(x) Very small parties have a field day and can dictate the terms of post-election negotiation and wield disproportionate power in determining and shaping policies. While it is important to be fair to small parties, it is not correct for the tail to wag the dog as they hold the balance of power. They become king makers and this can sharply misrepresent the

will of the people; 

These are the known risks with Sachs C, especially with a relatively high number of PR seats and a low eligibility threshold. It converts the mixed FPTP/PR formula into a pure PR model. A simulation of the 1987 general elections in Mauritius points in the same direction as what happened in Lesotho in 2012. A clear FPTP winner would have been transformed into a hung Parliament after the allocation of PR seats through the compensatory formula. Of course it appears fair to all parties. But then, proportionality is only one feature of a good electoral system, arguably not even the most important one. Countries such as the UK, France, USA, Canada, India and Australia, which are all very deeply democratic countries, rank stability well ahead of proportionality. Sachs C could pose a serious threat to what many consider the most important criteria of an electoral system. Stability, governability and decisiveness have, by and large, been the hallmark of our FPTP system since Independence. The population expects a winner once the votes are counted ! This will not be the case with Sachs C in many elections.

Confusion Three : Some reckon that 20 PR seats with no legal threshold is good. Not true at all. Confusion Four : Some want a legal threshold of 5%. It looks fair but it could rock the system.

There is an ongoing debate on the threshold to be entitled to PR seats. Some have recommended a natural threshold with 20 PR seats and without a legal impediment. The idea is that a natural threshold of 5% will automatically apply so as to ensure that each share of 5% of national vote is rewarded with one PR seat (100 divided into 20). This is true only if the allocation of seats is done through the parallel mode that distributes PR seats in total disregard of FPTP constituency outcomes. However,the parallel method has been comprehensively rejected by almost everyone as it does not cure the huge distortion between vote share and seats share. Others have suggested a low legal threshold of 5% to be fair to small parties.

If instead the alternative compensatory formula is used to apportion the 20 PR seats, then the picture changes drastically. This must be fully understood before we make an informed choice on the right threshold. With 62 FPTP seats and 20 PR MPs, the natural threshold (as opposed to the legal one) would be significantly lower than 5%. In fact, parties with around 1.2% of the national votes will receive one seat (100 divided into 82 as Sachs C would do it). In truth, if the Sainte-Lague highest average method is used (as in New Zealand) instead of the D’Hondt formula (as in Rodrigues), even a party with less than 1% of national vote will capture one seat under Sachs C !. This would lead to a profusion of parties and a complete fragmentation of the political system. Is this what the country wants ? .

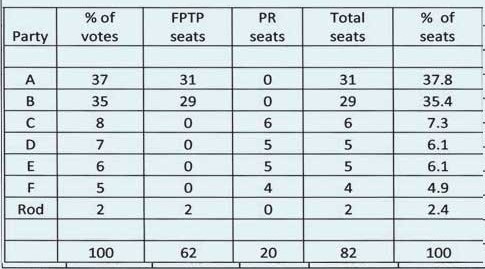
Even a 5 % legal threshold poses a major threat to the stability and effectiveness of the mixed system in Sachs C. I have simulated the election results with six parties in the island of Mauritius and two in Rodrigues. The results are shown in the table below.

This is a classic case of two major parties and four smaller ones that each obtains at least 5% of national votes. Not far from what could be expected in the future. I have assumed 62 FPTP and 20 PR seats for the sake of illustration. The main findings are as Follows

i) the two major parties capture the 60 FPTP seats in the island of Mauritius. Party A polls 37% of the national votes and takes 31 constituency seats while Party B captures 29 FPTP seats on 35% of the votes. One of the two parties in Rodrigues wins the two constituency seats there;

ii) six parties in total obtain at least 5% of national votes, including the two major ones that take the 60 FPTP seats. Parties C, D, E, and F garner 8%, 7%, 6% and 5% of the national votes respectively;

iii) the 20 PR seats are allocated through the compensatory formula as proposed by Sachs C. As it considers the totality of the seats and is highly proportional, it does not award any PR seats to Party A and Party B. They have already obtained their fair share of the total seats in the constituency contests (37% of 82 represents 30 seats while 35% of 82 gives 29 seats and both have their deemed percentage of seats already with 31 and 29 respectively );

iv) all the 20 PR seats go to the four smaller parties based on their percentage of national votes. Parties C, D, E and F receive 6, 5, 5 and 4 PR seats respectively;

v) the overall results of the mixed FPTP/PR system are extremely proportional. Party A has 37.8% of the seats on 37% of the votes while Party B takes 35.4% of the seats with 35% of the votes. The same trend applies to the other four smaller parties;

vi) had we adopted a pure PR voting formula with 82 list seats, the results would have been identical to the one above, except for the two seats in Rodrigues. This shows that there is no difference between a mixed FPTP/PR system that allocates seats through Sachs C and a full list PR formula;

vii) just like in Lesotho, we end up with a hung Parliament without a clear winner. Postelection negotiations are necessary and the small parties will have disproportionate power and will become king makers;

viii) it should be clear that we are not speaking about 1 seat for 5% of the votes. It is a completely different game altogether. All the 20 seats accrue to small parties and none to the larger ones. An innocuous proposal that appears reasonable however leads to very complicated electoral consequences. This is political fragmentation at its worst with potentially catastrophic implications for stability, governability and effectiveness. Is this what the country wants?

Concluding Remarks

The mathematics of electoral system may be uninteresting and complicated. However it is extremely important for all political and civil society actors to fully grasp the mathematical significance of each proposal so that the country makes the decision with its eyes, its ears and its minds open. And we should avoid both unintended consequences and unpleasant surprises. The country should not get more than it bargained for. These are undisputed facts and incontrovertible figures and not opinions where there could be divergence.

First, if some stakeholders want a very weak remedy that is similar to ‘panadol to cure cancer’ and which would keep the huge disproportionality between vote share and seat share, then they should be clear between the parallel and the compensatory mode of distributing PR seats. The difference between the two formulae is simply a chasm in terms of fairness and equity on the one hand and stability on the other. The parallel formula is very ineffective in producing a fair outcome while the compensatory mode is too proportional at the expense of stability. This is simple arithmetic.

Second, if some actors want a pure PR system for Mauritius, then they should make it very clear to the population that this is what they are proposing and not pretend that a mixed FPTP/ PR electoral system using Sachs C is different from a full PR formula. It is plainly not true. They are the same in the overwhelming majority of cases. This is straight mathematics.

Third, those who are honestly advocating a low threshold should recognise its implications when seats are alloted through Sachs C. It is not a question of one seat for each 5% of votes. It could be the totality of the 20 PR seats going to small parties thus giving them a disproportionate hinge power in the negotiation and in policy making. While the elephant should not bully a small animal, it is unfair for the small animal to hold hostage the elephant ! Again this is plain mathematics.

To overcome these three daunting challenges, I have proposed the use of an alternative formula to distribute PR seats among eligible parties, based on ‘Wasted Votes’. Of course, it is not an ideal formula but it has three key advantages. First, as it sits between Sachs A and Sachs C, it is much better than the parallel mode in terms of fairness and superior to the compensatory system in terms of stability. Second it does not convert the electoral system into a pure PR formula and it will thus provide a winner even in closely contested elections while being fair to unsuccessful parties. And third, it will mitigate the fragmentation of the party system as the basis of seat allocation is different from Sachs C. In essence, it is a good balance between the two most important values of a good electoral system. Stability and fairness. This is equivalent to a student that scores 90% in one important subject and 10% in another equally key subject ( Sachs A) and another one that does exactly the reverse with a score of 10% in the first subject and 90% in the second (Sachs C). The alternative recommended probably records around 75% in both subjects. This is the plain reality. It is up to us to decide what is least ‘harmful’ of the three formulae.

As should be manifestly clear from the avalanche of proposals being made, it is not an easy task to choose the right electoral system in a plural society. However it is important to distinguish between facts and fiction, dream and reality and above all between a deep understanding of the mathematical and policy implications of all proposals and a cursory feel based on superficial insight. The electoral system is too important a cog in the democratic wheel of our society to afford the luxury of believing that different things are similar and that similar things are different. And that small must be protected at any cost.. This is undoubtedly the case with the confusion between Sachs A and Sachs C on the one hand and between Sachs C and Sachs D on the other. This equally applies to the proposal to have 20 PR seats with a natural threshold or one with a legal threshold of 5%. We need a balancing act between stability and fairness and not a one sided formula that will rock the boat and erode the effectiveness and decisiveness of the system. One can only hope that the country will benefit from a more informed, balanced, enlightened and dispassionate debate. With an enhanced understanding of the mathematics of electoral reforms.

It is important to fully grasp the mathematical significance of each proposal so that the country makes the decision with its eyes, its ears and its minds open.